CS 39R: Symmetry & Topology
Lecture #4 -- Mon. 2/25, 2019.
PREVIOUS <- - - - > CS 39R HOME < - - - - > CURRENT < - - - - > NEXT
Preparation:
Design
a genus-6 object of high symmetry: Can you do this
with "spherical" symmetry {chart
2} --?
Present your design as a physical model, using: clay, paper,
styro-foam, pipe cleaners, . . ., or as a
computer model; or as a very nice drawing.
Be prepared to tell us what its symmetry is.
Warm-up:
Discuss your genus-6
object and its symmetries with your neighbors.
If you all come to an agreement, try to find a
general formula that
specifies the maximal amount of symmetry that a
handle-body of genus g may have.
Review the Symmetry Charts.
Brief Review of the 14 Symmetry Families of Compact (of finite
size) 3D Objects:
Check the posters on the wall and on the these two
charts:
"Chart
1": 7 families of rotational groups based on the 7
friezes wrapped around a cylinder: Cn, Dn, S2n, Dnd, Cnh, Cnv.
Dnh.
"Chart
2": 7 Types of "really 3D" symmetries based on the
Platonic and Archimedean solids.
Visualization of Symmetry Groups Using Shape Generator Programs:
Jane Yen and C. H. Séquin: "Escher Sphere
Construction Kit" Presentation at I3D conference
(PPT)
The Topology of Handle-Bodies (A Key
Concept!):
Examples of simple "handle-bodies":
(g=1; g=2; g=3; g=7):
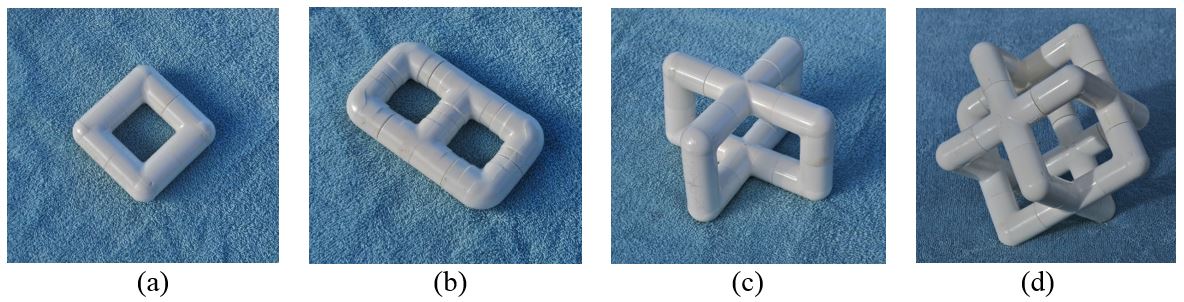
These are all equivalent definitions of "genus"
(in this particular context):
How many tunnels are there through this (volumetric)
body?
How many handles are attached to a simple spherical
blob?
(How many
"arms" need to be cut so that there are no longer any loops, but
the object is still connected?)
How many closed boundary loops can be drawn on this
surface that do not yet partition it into separate regions?
(You can still
go from any point to any other point without crossing any of
those boundary loops).
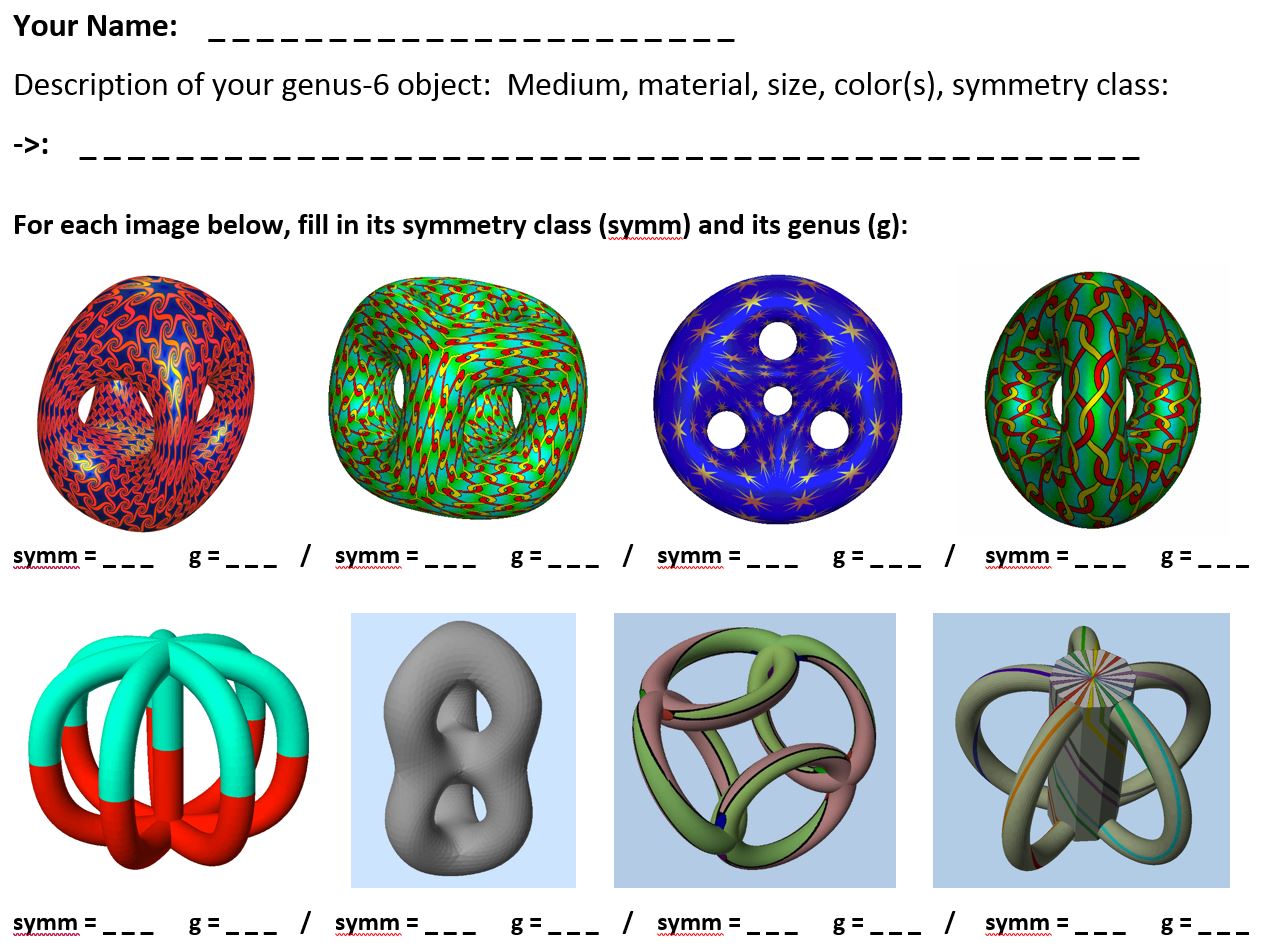
Worksheet: The Genus and Symmetries of
Handle-bodies.
Introducing "2-Manifolds" (Another Key
Concept!):
The surfaces of the
above handle-bodies are 2-manifolds.
A 2-manifold is a mathematical surface where
every small neighborhood has the connectivity of a small disk,
(or half-disks, if the surface has holes and borders);
but no "multi-leaf" branching as at the spine of a book!.
Surfaces of handle-bodies happen to be
orientable and 2-sided:
They have an "inside" towards the material of the body and an
"outside" towards the surrounding air.
From now on, we focus on this infinitely thin mathematical
surfaces.
So far these surfaces were free of "holes" or "punctures."
Introducing "Punctures":
If a small disk-like patch is removed
from a 2-manifold, its genus remains the same;
but it now has a "puncture" with a "border".
A thin spherical shell with n holes is still a genus_0
surface, but one with n punctures (with n
borders).
Note: Do not confuse this with drilling tunnels
into a thick-shelled, hollow (volumetric) spherical ball!
A single such tunnel into the inside of a thick-walled sphere
results in a "fat disk" -- which is a lump of genus 0.
Drilling t such tunnels into a thick-walled sphere turns
this into a volumetric object with a surface of genus t-1.
(Asking for the genus of a thick-walled sphere without any
tunnels, is not appropriate, because it has TWO surfaces: inner
and outer!)
Basic examples:
Sphere surface: Genus = 0; NO punctures (# of
borders = 0)
Simple disk: Genus =
0, # of borders = 1 (the outer rim). This is the
same as a sphere with one (big) puncture!
Something you will NOT find as a surface of a Handle-Body:
Single-sided, Non-orientable 2-Manifolds:
Moebius-Bands and Klein Bottles: PPT
What is a Moebius band?
Moebius bands come in many different forms...
These are NOT Moebius bands...
What is a Klein bottle?
Klein bottles also come in many different forms...
The Surface Classification Theorem: PPT
All
2-manifolds can be characterized topologically by just THREE
parameters:
its sidedness (1 or 2); its number of borders (0 ...
infinity); its genus (0 ... infinity).
==> To be continued ... See also:
Video: "From
Klein Bottles to Super-bottles"
New Homework Assignments:
Fill in the blanks in the Work-sheet
on Surface Classification
(Hand-out)
(This may be hard. Do as well as you can. Do not
despair. We will go over this in detail next lecture.)
Due: in class, March 4, 2019
Review
the concepts of 2-manifolds, Moebius-bands, and
Klein-bottles by studying this PPT and
the comments below the slides.
Preview
the concept of Surface
Classification, so you better prepared for an
in-class discussion.
And if you
feel comfortable with the above, also watch the video: "From
Klein Bottles to Super-bottles"
PREVIOUS <- - - - > CS 39R HOME < - - - - > CURRENT < - - - - > NEXT
Page Editor: Carlo H. Séquin