Misinterpretations and Mistakes in Pacioli's Rhombicuboctahedron!
Stimulated by the controversy caused by this note posted on Scientific American:
http://www.scientificamerican.com/article.cfm?id=davinci-mathematical-slip-up
which then led to my construction of this web page: http://www.cs.berkeley.edu/~sequin/X/Leonardo/leonardo.html
I also took a closer look at the rhombicuboctahedron depicted in the Pacioli painting.
Here is a discussion of this painting by Herman Serras. He states:
"The object is made of glass plates and it is half filled with water. The painter has captured the reflections and
refractions in an extraordinary way
and Mackinnon attributes this part of the ritratto to Leonardo da Vinci himself!"
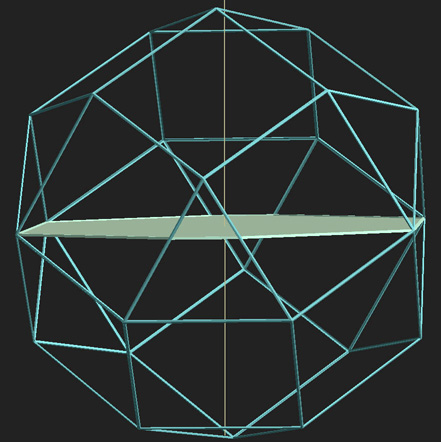
After creating a thin frame model of a rhombicuboctahedron, I tried to match as best possible the eye-(camera-)position
and the perspective zoom factor in the painting in order to make the analysis and discussion below easier to follow.
Two main facts emerge:
1.) This painting does not depict a glass polyhedron filled with water, and
2.) the depicted reflections are mostly imaginary, since they are quite wrong.

George Hart on his page devoted to Luca Pacioli's Polyhedra states:
"The
polyhedron in the painting is a masterpiece of reflection, refraction,
and perspective.
(Davis states that the bright region on its surface reflects
a view out an open window, showing the Palazzo Ducale in Urbino.)
Certainly an actual glass polyhedron was used as a model.
(Pacioli states
in his books that he constructed several sets of glass polyhedra, but I
know of no other information about them.)
The polyhedron in the painting
is beautifully positioned, suspended with a 3-fold axis vertical,
out of
physical contact with the other objects in the scene."
Suspending the polyhedron with a line from the middle of the
bottom triangular face through the middle of the top triangular face,
along the 3-fold symmetry axis of the
rhombicuboctahedron, leads to a contradiction:
The top and bottom faces would then be
perfectly level, and both of them are seen from their lower side.
Thus the view center (eye-level) must be below the polyhedron.
However, the water-surfac is seen from the top.
And indeed, when we look carefully, we can see that this central surface
coincides with 4 of the 24 vertices of the rhombicuboctahedron.
(It also intersects four more triangle edges at a ratio of 0.4142 (= sqrt(2) - 1) measured from the shared tip in the triangle).
If the polyhedron frame is now rotated about 90 degrees to the left
around its vertical axis, we can see clearly how much that plane is
slanted.
In order to see the water-surface from above, the RCO has to be tilted away from the viewer by about 10 degrees.
But this means that the suspension line (red) cannot go through the centers of the top and bottom triangles.
I discovered recently that Herman Serras has noticed this fact many years ago and has calculated the proper intersection points
between the suspension line and the two triangles: The location is 0.4142 (= sqrt(2) - 1) away from the tip towards the opposite side.

Perspective:
So which suspension hypothesis is correct? -- To answer that
question I took a closer look at the perspective in the painting:
Based on a best fit of my computer drawing, the perspective implies
that the object is seen from about 14 RCO diameters away.
Now based on its position and comparing it to the hands and head of
Pacioli, I would guess that it is about 8-9 inches in diameter.
Thus, based on its own perspective, it must have been drawn as it would
look from about 10 feet away .
But Pacioli in the painting seems only about 5-6 feet away from the
viewer, and the object, if it is above the table, would then be only 3-4
feet away.
Thus the perspective of the
rhombicuboctahedron is not compatible with the rest of the scene.
The RCO should exhibit a much stronger perspective!
I strongly suspect that this RCO was drawn quite carefully, but
separately, -- perhaps from a model or from a geometrical construction --
and subsequently copied into the Pacioli painting.
Nevertheless it can provide us with a resolution to the suspension
issue, if we analyse the viewer's eye level with respect to the
painting.
My own intuition, when looking at this painting, tells me that my eyes are about at the height of
Pacioli's nose or eyes,
and that the person on the right is looking slightly down
on me. This would place the view center properly above the water level.
I also tried to find a horizon compatible with the objects on
the table, and I found:
1.) a vanishing point on top of Pacioli's head for the slate tablet;
2.) a vanishing point somewhat above the middle of the RCO (but
way out on the left) for the open book (with substantial error margins);
3.) a vanishing point about half a head-height above the top of Pacioli's
head for the red box (also with substantial error margins).
All of these vanishing points are higher than the center of the RCO, and that explains why we see the water surface from above.
But then, the bottom triangle must be slanted upwards towards the viewer, so that we can see its lower side.
==> Thus the red suspension line wins. The water surface is indeed horizontal.
So, did the painter indeed observe a water-filled glass polyhedron? -- I don't think so!
The convincing clue is that there are no refractive effects visible in the "water-filled" bottom half.
The edges on the backside of the polyhedron appear almost precisely in
the locations to which the edges of my empty polyhedral frame are being
projected.
With water inside this object, those apparent edge positions would be moved dramatically because of refraction.
Thus, it may be possible that this internal surface is an
additional (irregular) octagonal plate,
which may have been inserted
into this glass shell to give it some stability, ( -- if indeed this object was ever actually built from glass plates!).
But it is more likely, that this is an artistic rendering of an imagined water surface inside a real, or imagined, glass model.
Reflections:
Do the depicted reflections imply that the painter was looking at a real glass object? -- I don't think so!
Nick Mackinnon in "The portrait of Fra Luca Pacioli", The Mathematical Gazette,
77 (1993)
states that there is a
reflection of a house in one of the bottom panels in the RCO.
And the bright reflection in the upper left (near "10-o'clock") supposedly (see above)
"...reflects
a view out an open window, showing the Palazzo Ducale in Urbino."
The SciAm posting referenced above (top of page 2) claims that:
"At the same time, the reflections of the light in and on the model are
marvellously drawn. This seems practically impossible without models."
Yes, it would indeed by very difficult to draw those reflections
correctly without an actual model -- or without a computer graphics program!
And, yes, perhaps they are "marvelously drawn" -- they are just not correct!
That reflection of that window is drawn like a "paper sticker" containing the
view from the window,
which has then been pasted onto two adjacent
facets of the polyhedron.
However, when the reflection of a rectangualr window stretches across an edge with a
substantial dihedral angle, the continuity of the reflection is broken,
and it is split apart into two quite different, dis-connected
reflections. Thus this must be a beautifully drawn reflection -- as
imagined by the artist!
The inside reflection on the lower right would also look quite different in the presence of water!
Marcel Tunnissen drew my attention to the dark person reflected in the front-facing triangle.
I suspect that this is the painter trying to represent himself in this painting.
However, since the RCO is turned slightly to the left, the
painter's reflection should not appear in the center of that triangle!
-- Yet another imaginary reflection!
More recently I discoverd an interesting posting by Joost Rekveld: light matters
in which he also discusses the reflections in the RCO and reaches the same conclusions.
He also provides a photograph that shows these reflections much more clearly.
. . . More and more intriguing mysteries surround this painting!
Page Editor: Carlo
H. Séquin



