Sculpture Designs by Carlo Sequin
Multiply Split Tori
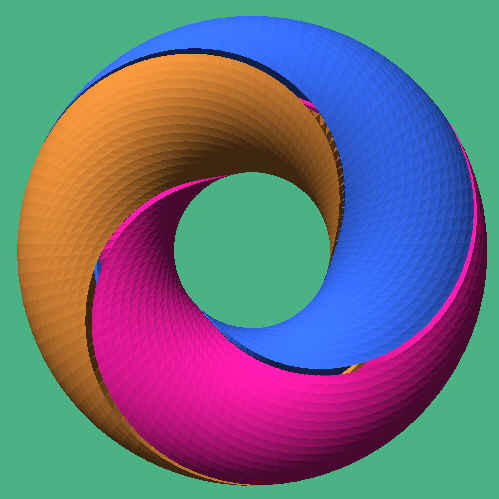
By making a "Y"-shaped cut and rotating it 360 degrees while traveling
around the toroid,
the torus can be split into three mutually interlocked parts.
Since the situation is somewhat on the congested side, when the central
hole is equal to the worm diameter,
I made another toroid with a hole twice as large, relatively;
I also changed the swept profile to a hexagon, (the individaul strands
now have a rhombic profile),
so that inner and outer surfaces have the same geometry and can thus
be matched up easily.
 --
--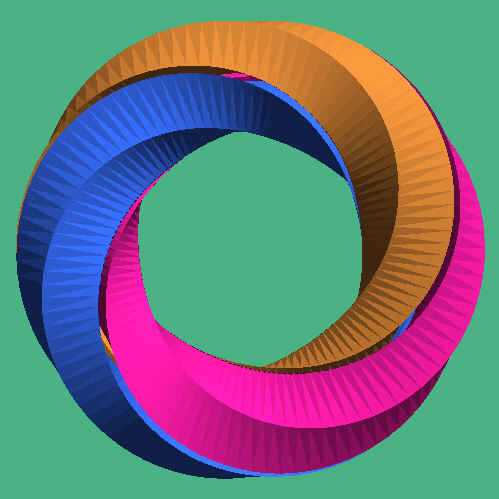
And these are the kind of configurations that you can make with these
three rings ...
 --
--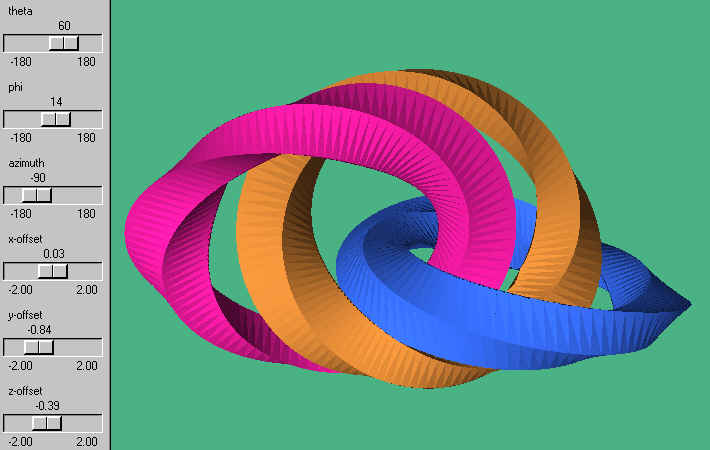
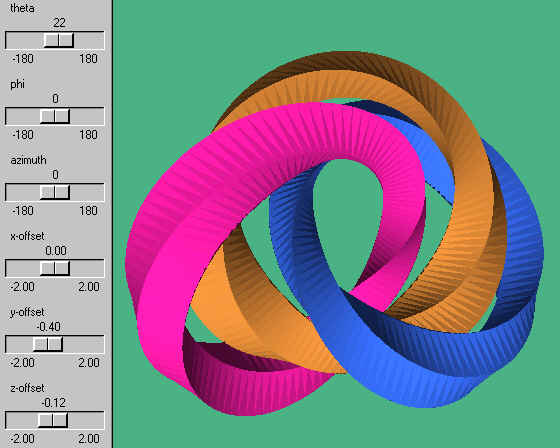
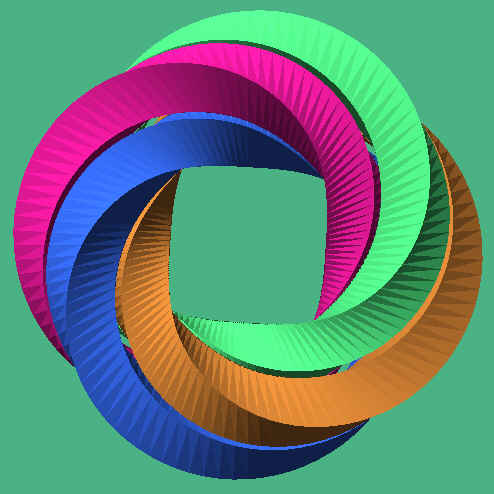
Here is an example of a torus split into 4 mutually interlocking rings:
Moebius Space
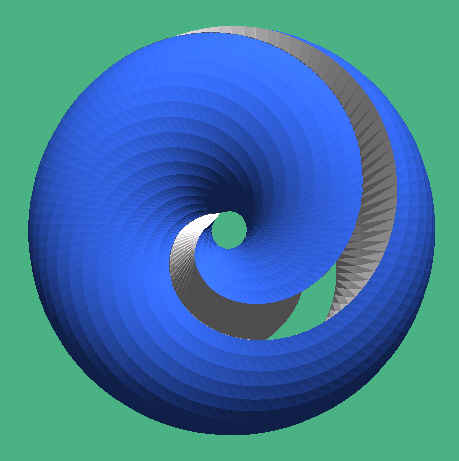
If the cutting swath traveling around the toroidal loop is twisted only
through 180 degrees, rather than 360 degrees,
it does not cut the torus into two parts, but splits it into a semi-circular
beam traveling around the toroidal loop twice
before it reconnects to itself. This is because the enclosed splitting
space is "single-sided", i.e., the same material is
on both of it side.
Nat Friedman suggested, that this empty Moebius space might itself
be made the key feature of some sculpture.
To that effect, the gap should be made wide enough so that one can
easily see inside. Also, the center hole
of the torus should be made rather small. So, Nat, these pictures are
for you ...
 --
--
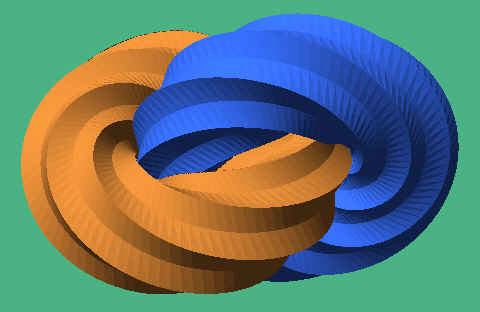
Interlocking Serrated Tori
I was experimenting with two tightly interlocking tori. To make this
tangle more interesting, I tried to find a way
to make serrated surfaces that would tightly interdigitate like the
teeth in a well-designed gear.
One way to do this, is to make longitudinal serrations on one torus,
and latitudinal ridges on the other.
But can the two parts be made identical ?
Below is a seven-tooth version. The two tori can actually move with
respect to one another
-- forming some kind of a pathological worm-gear.
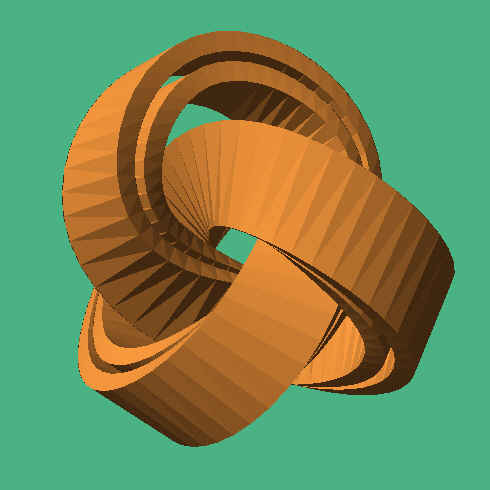
Split Trefoils
In this series, I start from a constructivist version of a trefoil knot.
By choosing suitable geometry of the generating path and the right
amount of twist,
the band can be made into a single-sided surface.
This Moebius trefoil band can now be split down its middle,
resulting in a band of half the width and twice the length,
that travels around the trefoil twice.
Alternatively, we can split the band in its thickness direction into
"two layers".
 --
--
With different parameter settings, the geometry can be modified
so that the overall twist is now an odd multiple of 90 degrees.
This allows us to split the knotted prism "twice" lengthwise
(it is really just a flat cut going around the knot twice,
or alternatively a cross-shaped cut going around once).
This results in a single prismatic beam, traveling around the knot
four times.
 Here is a bigger picture, including my user interface.
Here is a bigger picture, including my user interface.
Back to the KEIZO
USHIO Page
 --
--
 --
--

 --
--

 --
--
 Here is a bigger picture, including my user interface.
Here is a bigger picture, including my user interface.