CS 39R: Symmetry & Topology
Lecture #4 -- Mon. 2/22, 2016.
PREVIOUS <- - - - > CS 39R HOME < - - - - > CURRENT < - - - - > NEXT
Preparation:
Design
a genus-6 object of high symmetry:
Present your design as a physical model, using: clay, paper,
styro-foam, pipe cleaners, . . ., or as a
computer model; or as a very nice drawing.
Be prepared to tell us what its symmetry is.
Warm-up:
Discuss your genus-6 object and its
symmetries with your neighbors.
If you all come to an agreement, try to find a
general formula that
specifies the maximal amount of symmetry that a
handle-body of genus g may have.
Brief Review of the 14 Symmetry Families of Compact (of finite
size) 3D Objects:
Check the posters on the wall and or the these two
charts:
7
families of rotational groups based on the 7 friezes wrapped
around a cylinder: Cn, Dn, S2n, Dnd, Cnh, Cnv. Dnh.
7
groups of "really 3D" symmetries based on the Platonic and
Archimedean solids.
The Platonic Solids:
Tetrahedron, cube (hexahedron), octahedron,
dodecahedron, icosahedron.
Can you explain to a high-school student why there are exactly
five Platonic Solids
?
A more complicated structure:
It has oriented icosidodecaheral symmetry; no mirror planes.
Visualization of Symmetry Groups Using Shape Generator Programs:
Jane Yen and C. H. Séquin: "Escher Sphere
Construction Kit" Presentation at I3D conference
(PPT)
The Topology of Two-sided, Orientable
2-Manifolds:
A 2-Manifold is a mathematical surface where every
small neighborhood has the connectivity of a small disk,
(or half-disks, if the surface has holes and borders);
but no "multi-leaf" branching as at the spine of a book!.
Examples of simple "handle-bodies":
(g=1; g=2; g=3; g=7):
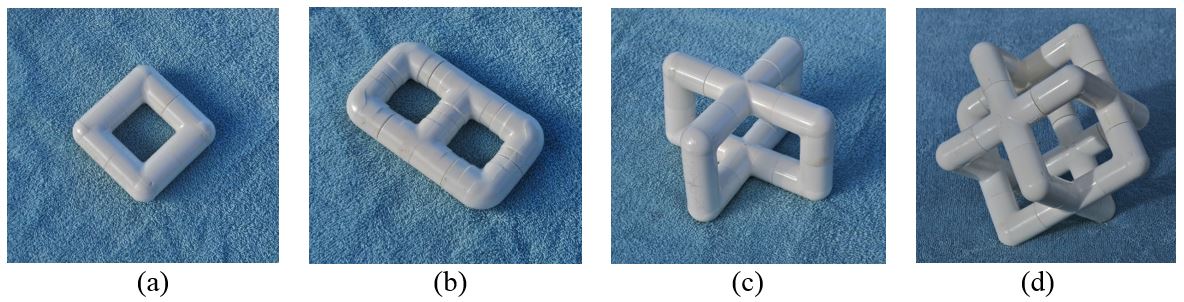
These are all equivalent definitions of "genus"
(in this particular context):
How many tunnels are there through this (volumetric) body?
How many handles are attached to a simple spherical blob?
(How many
"arms" need to be cut so that there are no longer any loops, but
the object is still connected?)
How many closed boundary loops can be drawn on this surface that
do not yet partition it into separate regions?
(You can still
go from any point to any other point without crossing any of
those boundary loops).
Introducing "Punctures":
If a small disk-like patch is removed
from a 2-manifold, its genus remains the same, but it now has a
"puncture" with a "border".
A spherical shell with n holes is still a genus_0
surface, but one with n punctures (with n
borders).
Note: Do not confuse this with drilling tunnels into a
thick-shelled, hollow (volumetric) spherical ball!
A single such tunnel turns the thick-walled sphere into a "fat
disk" -- which is a lump of genus 0.
Drilling t such tunnels into a thick-walled sphere turns
this into a volumetric object with a surface of genus t-1.
(Asking for the genus of a thick-walled sphere without any
tunnels, is not appropriate, because it has TWO surfaces: inner
and outer!)
Worksheet: The Genus, Borders, and
Symmetries of Orientable Handle-bodies!
The Topology of Single-sided, Non-orientable
2-Manifolds: PPT
What is a Moebius band?
Moebius bands come in many different forms...
These are NOT Moebius bands...
What is a Klein bottle?
Klein bottles come in many different forms...
The Projective Plane and Boy's Model.
Two Moebius bands together make a Klein bottle:
Limerik;
Demo with Cliff Stoll's zippered model.
How many different Klein bottles are there?
How to make a single-sided, non-orientable surface of
genus g ?
==> Graft g cross-caps onto a sphere.
"Cubist" Models of 2-Manifolds:
Compose the
surface using only planar facets parallel to one of the 3
major coordinate planes!
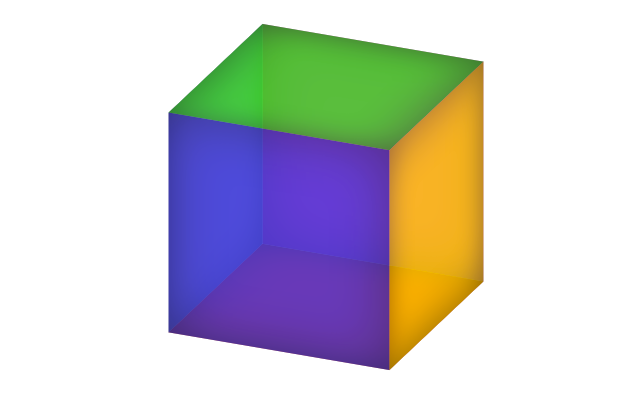
A
"cubist" sphere is a
cube.
A "cubist" donut is a "rectilinear picture frame".

New Homework Assignments:
Due: Feb. 29, 2016
Design a
"cubist" Moebius band: Only planar facets parallel to
one of the 3 major coordinate planes!
Present your design as a physical model, using: paper,
cardboard, plexi-glass, alu-foil, sheet-metal, or other
similar thin, flat materials.
Try to find a design with a minimal number of individual
facets, and (of secondary concerns) with maximal symmetry.
If it takes you less than 30 minutes to find a solution, try
to model a "cubist" Klein-bottle.
Bring your models to class on Feb. 29.
PREVIOUS <- - - - > CS 39R HOME < - - - - > CURRENT < - - - - > NEXT
Page Editor: Carlo H. Séquin

