CS 39R: Symmetry & Topology
Lecture #5 -- Mon. 3/4, 2019.

PREVIOUS <- - - - > CS 39R HOME < - - - - > CURRENT < - - - - > NEXT

Preparation:

Fill in the blanks in the Work-sheet on Surface Classification

Review the concepts of 2-manifolds, Moebius-bands, and Klein-bottles by studying this PPT and the comments below the slides.

Preview the concept of Surface Classification, so you are better prepared for an in-class discussion.

Warm-up:

What is the genus of the following handle-bodies:
-- A hollow thick sphere with 17 tunnels through its thick shell ?
-- A hollow sphere-shell with 17 internal spokes converging at its centroid ?
-- A cube frame with 8 diagonal spokes connecting the cube vertices to the centroid ?
-- A fully connected graph with thick tubular edges connecting 6 nodes ?
-- and the following depicted objects:

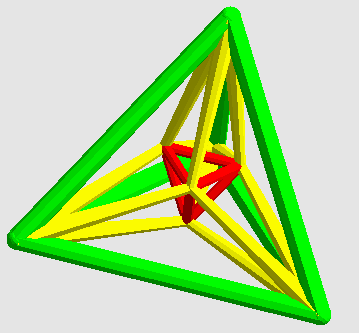
(tetrahedral symmetry) (12 4th-order saddles in loop) (24 monkey-saddles in trefoil)

When you are done, look at some other models (on the table) and practice your skills in finding symmetry axes
and determining the genus and the symmetry classification of these geometrical shapes.

This is hard work! Many confusing issues!
What is the connection between Genus and Euler Characteristic?
Let's relax and look at some simple but very important 2-manifolds:

Moebius-Bands and Klein Bottles: PPT

Now let's take a different approach and look at these surfaces (and some other ones) again in a "constructive" approach:

The different "universes" of 2D video games:

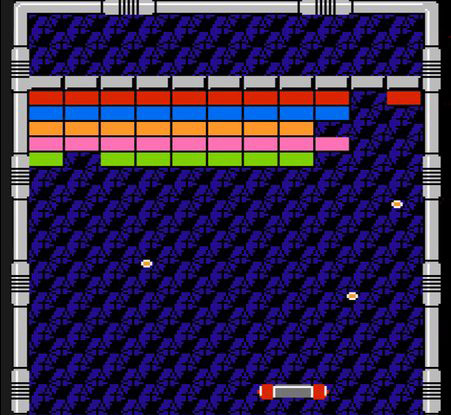
What happens to rockets or bullets that drift off to the perimeter of the screen?
How do the screens wrap around and connect to themselves?

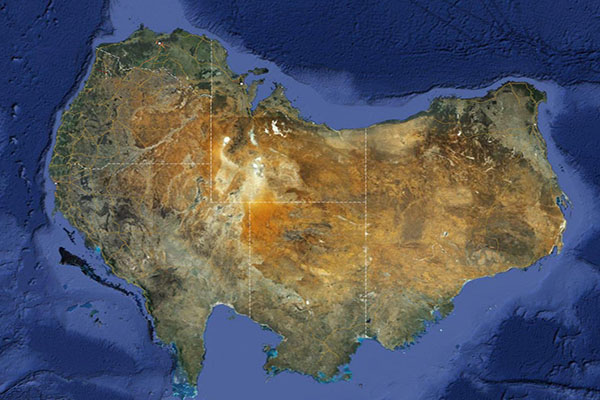
What might be the 2-manifold topology of the 2D-universe surrounding this island?
Discuss possible connectivities ("around the back-side") of this world.

Think of all possible (2-manifold) universes
that can be formed by connecting the edges
of a rectangle in different ways,
i.e., by identifying pairs of points on them
(assuming them to be connected).

Constructing simple topological 2-manifolds, -- orientable and non-orientable: PPT

Cylinders, Tori, Möbius bands, Klein bottles, Boy surface ...
They all have different surface classifications!

==>> DONE through here ... CONTINUE next time.

Surface Classification: PPT

For the topological classification of a 2-manifold, we need to count its border-loops, determine its sidedness,
and calculate its genus. The last task is the hardest one.
Often it can be solved most easily by calculating the Euler characteristic.

Euler Characteristic and Genus: PPT

Back to the Warm-up problems ...
A little bit of Graph Theory: "nodes" and "edges"
Make tree-like graphs without loops. How many edges do we need to cut to get there?
A final tree with n vertices has how many edges?

The above techniques should now allow you to calculate the genus even of very complicated 2-manifolds!

New Homework Assignment:

Due: March 11, 2019

1.) Fill in the blanks in a new Work-sheet on Surface Classification ==> "Learning by doing" !!

Some hints: The 4 structures in the bottom row are all basically a (twisting) ribbon knotted into a trefoil knot,
with some additional connections added.
In the pink trefoil the added connections are "twisting the other way" from the way they do in the grey trefoil.
(This is also a good exercise to train your brain to see and understand 3D objects from limited information.)

Bring the filled-in worksheet to class on Monday, March 11, 2019.

2.) Construct a physical model of a single-sided surface of genus 2 (e.g., using paper strips),
and then draw onto this surface two closed loops that together still leave the surface fully connected (just ONE domain).

Bring this model to class on Monday, March 11, 2019.

NOTE:
The new homework refers again to the "elusive" Euler Characteristic (EC).
When I prepared it, I did not consider that I might not get through all the material that I had planned for yesterday's class.

Please, on your own, study the PowerPoint presentation : "Euler Characteristic and Genus: PPT".
The first six slides should just be a pictorial review of what we have discussed in class.
Then, on slide #7 is the formal introduction of EC in the context of a polyhedral object
or of a mesh of tiles painted on the surface of a handle-body: EC = X = V - E + F.

At the bottom of the slide, there is a formula that relates EC (=X) and the genus on the handle-body,
taking into account the number of borders (b) and the sidedness of a surface
(in case it is not just the surface of a handle-body, but rather a Moebius band or a Klein bottle.)
This formula woks in both directions: If you know the EC, you can figure out the genus,
and if you know the genus, you can calculate the EC.

Slides #8 through #16 show the application of the above definition and formula to various examples.
This should help you to find the answers for "EC = __" for many problems.
(It even gives the explicit answer to one of the problems on your handout!)

Of course, we will go through all of this in our next class. Have your questions ready!

PS:
Also try to construct a single-sided paper surface of genus 2, showing the two closed (non-intersecting) loops
that demonstrate that this is indeed a genus-2 surface.